similar shapes and congruent shapes

Contributed by:

The Tutoring Experts Sun, Feb 26, 2023 05:51 PM UTC

Enlargements create shapes that are similar. When two shapes are the same in shape and size, they are congruent shapes. When two shapes are different in size but proportionally the same shape, they are similar shapes.

1. Name: ___________________________
GCSE (1 – 9)
Similar Shapes
Instructions
• Use black ink or ball-point pen.
• Answer all questions.
• Answer the questions in the spaces provided
– there may be more space than you need.
• Diagrams are NOT accurately drawn, unless otherwise
indicated.
• You must show all your working out.
Information
• The marks for each question are shown in brackets
– use this as a guide as to how much time to spend on each
question.
Advice
• Read each question carefully before you start to answer it.
• Keep an eye on the time.
• Try to answer every question.
• Check your answers if you have time at the end

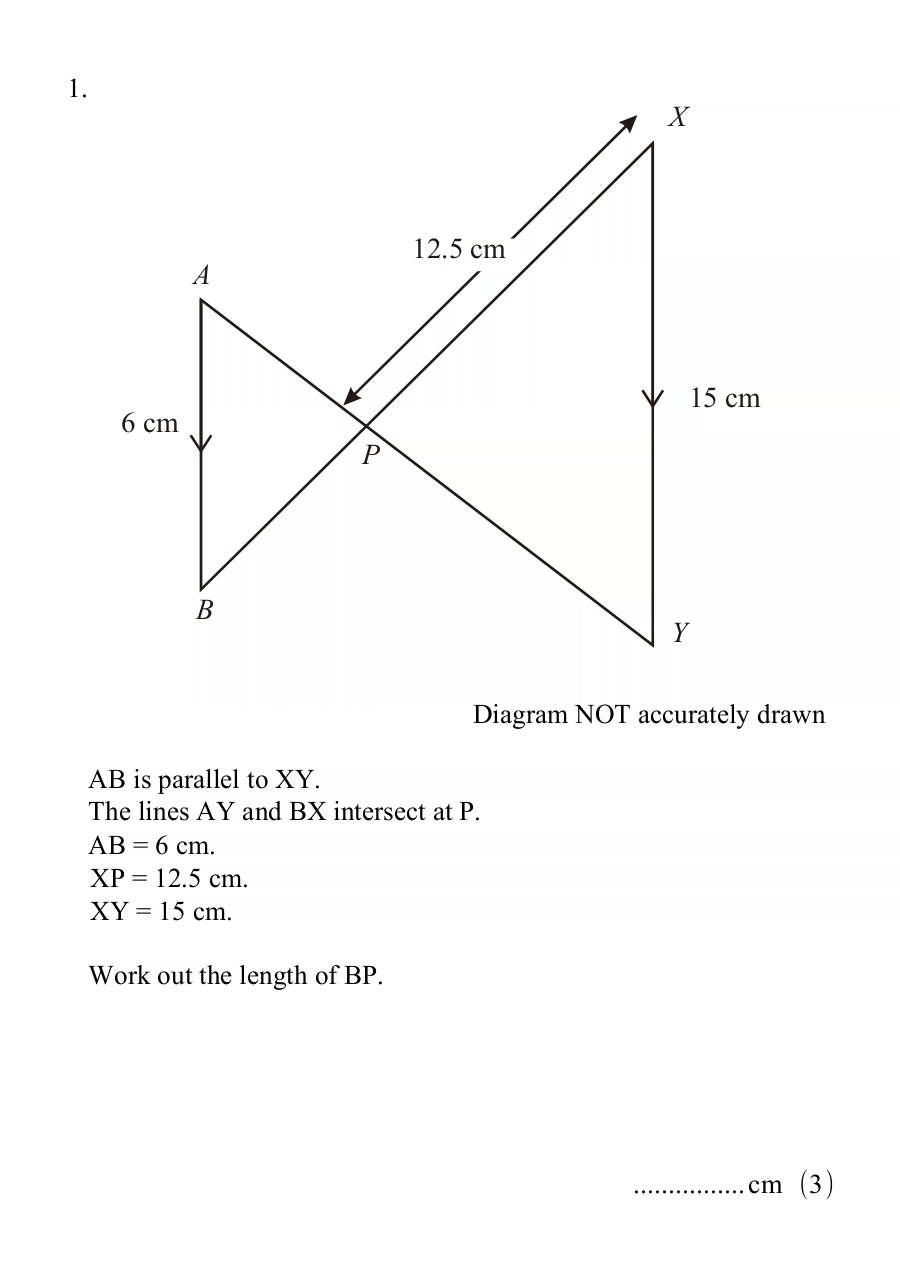
2. X
12.5 cm
A
15 cm
6 cm
P
B
Y
Diagram NOT accurately drawn
AB is parallel to XY.
The lines AY and BX intersect at P.
AB = 6 cm.
XP = 12.5 cm.
XY = 15 cm.
Work out the length of BP.
................ cm (3)

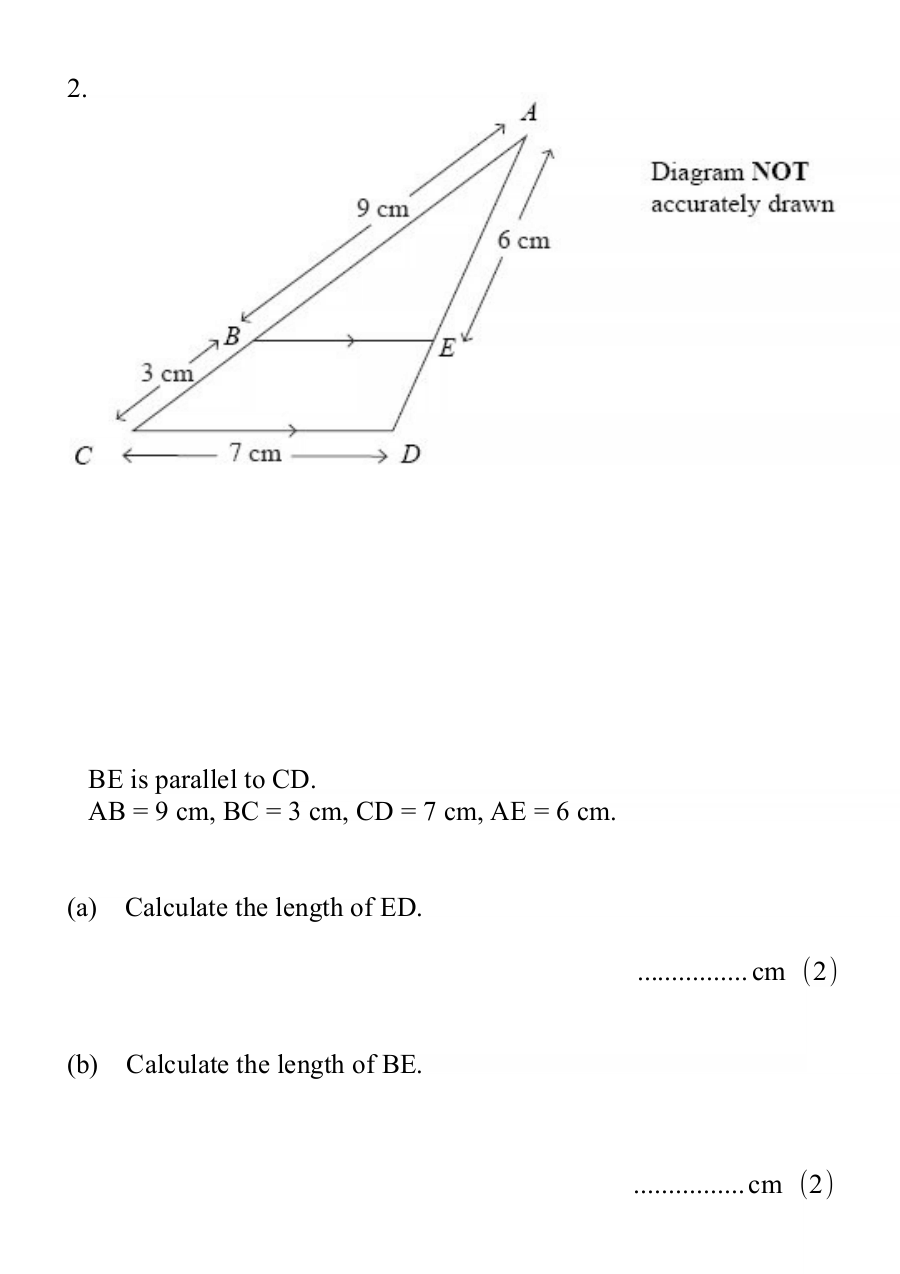
3. BE is parallel to CD.
AB = 9 cm, BC = 3 cm, CD = 7 cm, AE = 6 cm.
(a) Calculate the length of ED.
................ cm (2)
(b) Calculate the length of BE.
................ cm (2)

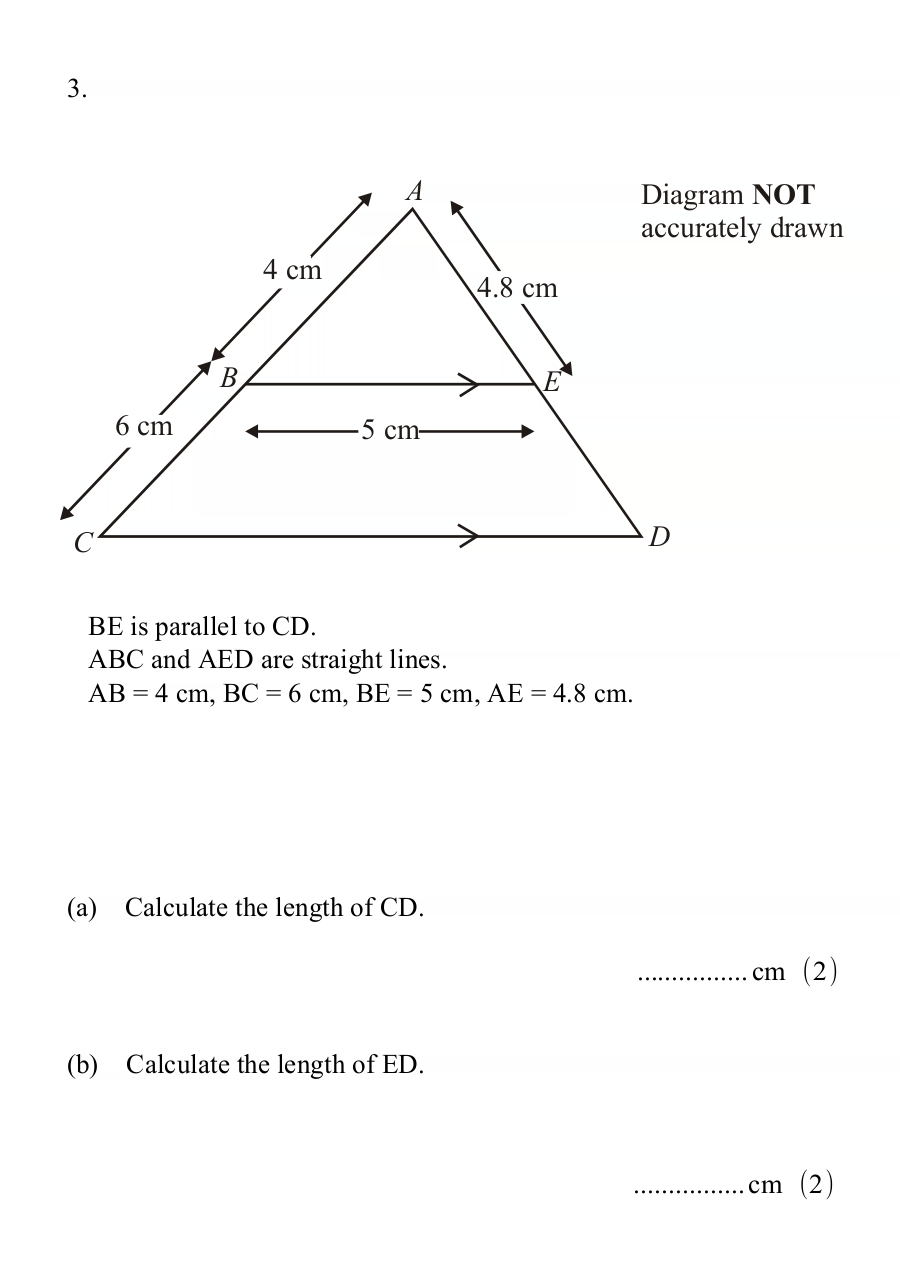
4. A Diagram NOT
accurately drawn
4 cm
4.8 cm
B E
6 cm 5 cm
C D
BE is parallel to CD.
ABC and AED are straight lines.
AB = 4 cm, BC = 6 cm, BE = 5 cm, AE = 4.8 cm.
(a) Calculate the length of CD.
................ cm (2)
(b) Calculate the length of ED.
................ cm (2)

5. 4.
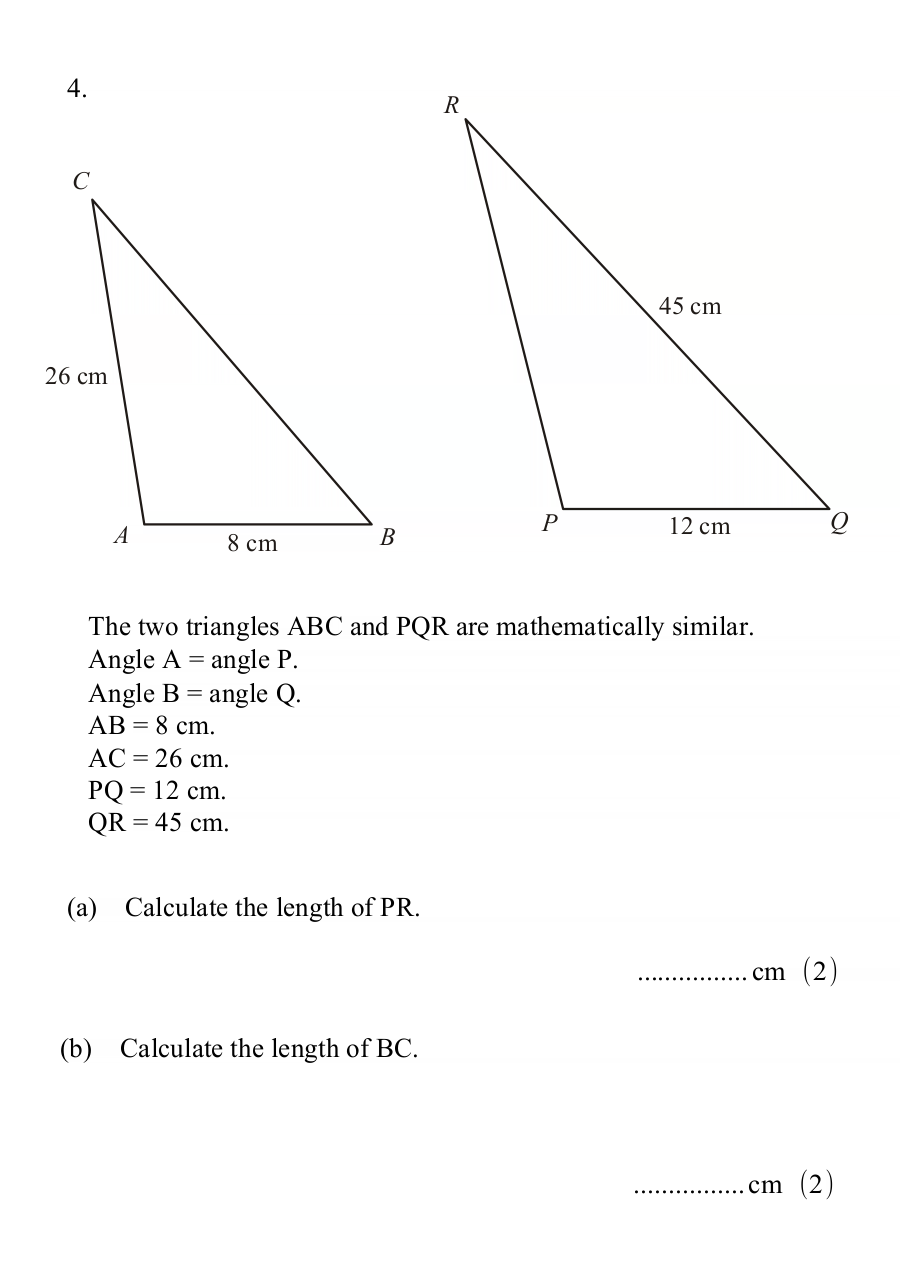
R
C
45 cm
26 cm
P 12 cm Q
A 8 cm B
The two triangles ABC and PQR are mathematically similar.
Angle A = angle P.
Angle B = angle Q.
AB = 8 cm.
AC = 26 cm.
PQ = 12 cm.
QR = 45 cm.
(a) Calculate the length of PR.
................ cm (2)
(b) Calculate the length of BC.
................ cm (2)

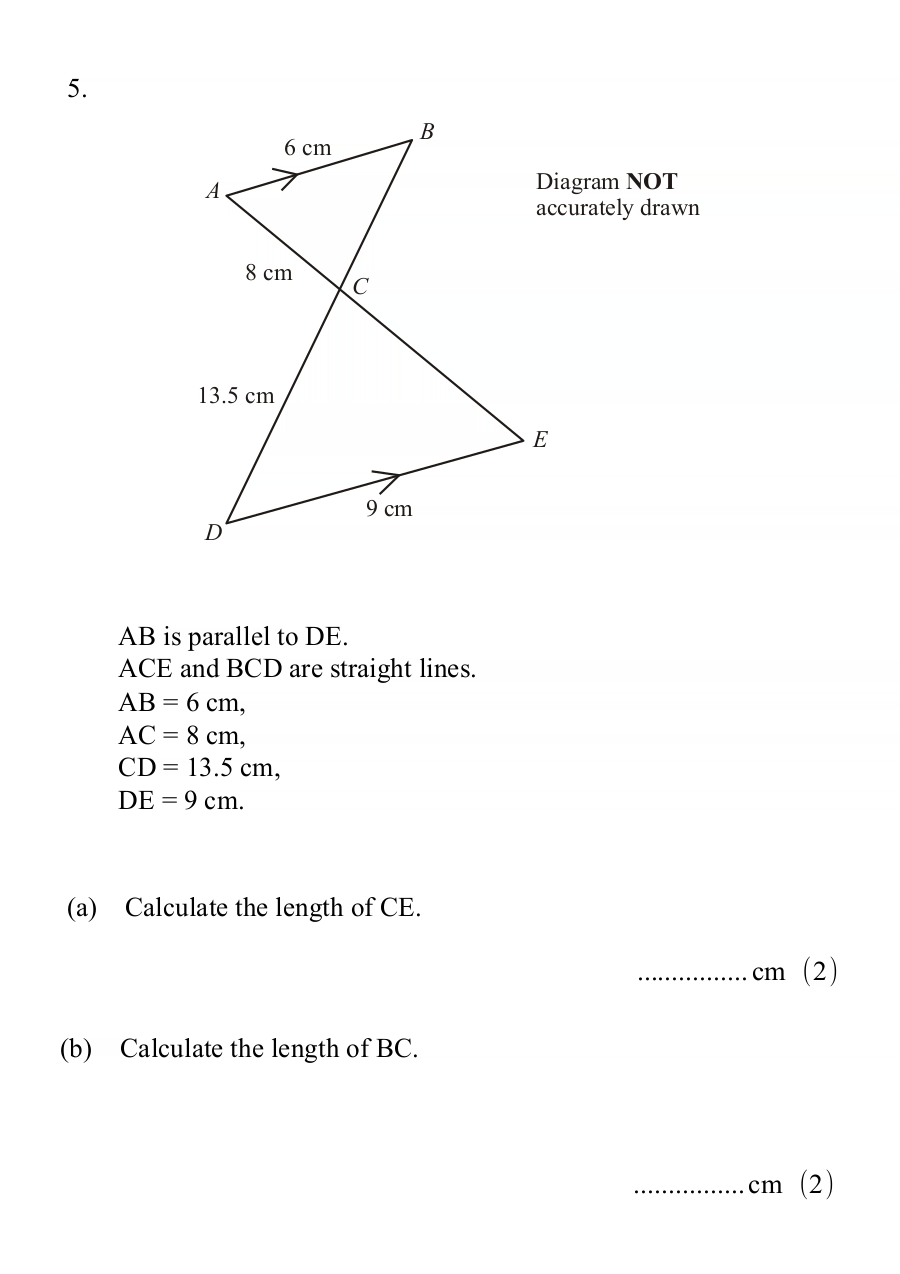
6. B
6 cm
A Diagram NOT
accurately drawn
8 cm
C
13.5 cm
E
9 cm
D
AB is parallel to DE.
ACE and BCD are straight lines.
AB = 6 cm,
AC = 8 cm,
CD = 13.5 cm,
DE = 9 cm.
(a) Calculate the length of CE.
................ cm (2)
(b) Calculate the length of BC.
................ cm (2)

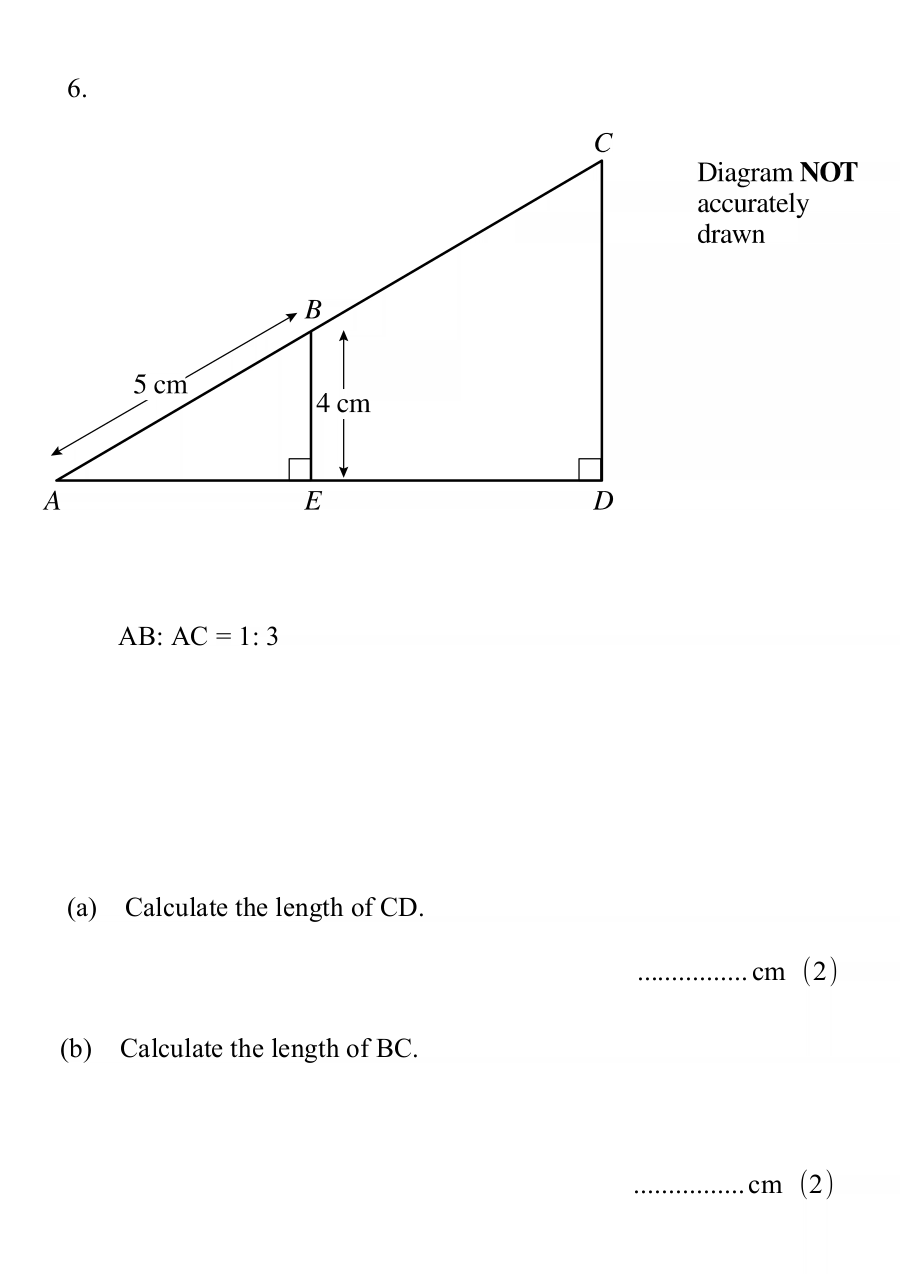
7. AB: AC = 1: 3
(a) Calculate the length of CD.
................ cm (2)
(b) Calculate the length of BC.
................ cm (2)

8. A 20 Euro note is a rectangle 133 mm long and 72 mm wide.
A 500 Euro Note is a rectangle 160 mm long and 82 mm wide.
Show that the two rectangles are not mathematically similar.
(3)

Book a Trial Class